Motivation

Figure 1: The Oakland-San Francisco Bay Bridge in the earthquake-prone area of California
incoherence effect, which represents random differences in the amplitudes and phases of seismic waves due to reflections and refractions during wave propagation and due to the differential superposition of waves arriving from different parts of an extended source; the wave-passage effect, which describes the differences in the arrival times of waves at separate locations; and the site-response effect, which accounts for differences in the intensities and frequency contents of surface motions due to variable soil profiles underneath the supports.
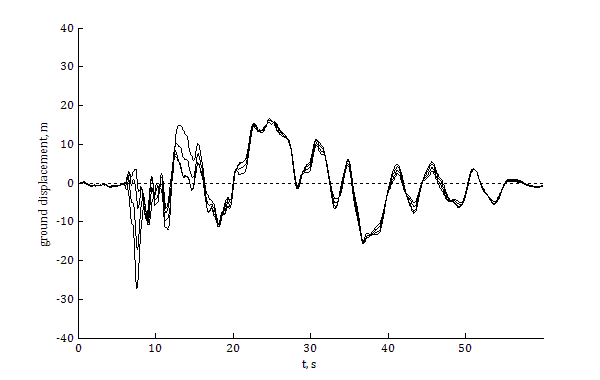
Figure 2: Effect of incoherence on seismic ground displacements
Under spatially varying support excitations, the response of a bridge can be expressed as the sum of two components: a pseudo-static component and a dynamic component. The pseudo-static component, which is zero in the case of uniform support excitations, is the static response of the bridge to the differential support displacements prescribed by the spatially varying ground motions at each time instant. Under uniform soil conditions, the effects of incoherence and wave passage tend to decrease the dynamic response due to random cancellations. However, by inducing a pseudo-static response component, these effects may increase or decrease the total bridge response, depending on the structural characteristics and the ground motion field. Thus, even in the case of uniform soil conditions, accounting for the spatial variability of ground motion is important. The additional consideration of the differential site-response effect tends to increase the pseudo-static component, whereas the influence on the dynamic component depends on the types of soils considered. In all cases, neglecting the spatial variability of ground motion may or may not be on the safe side. Thus, incorporating all three effects of incoherence, wave passage and site response in the earthquake response analysis of bridges is necessary.
A thorough investigation of the response of bridges to differential support excitations is performed employing response spectrum and time-history analysis methods. Response spectrum methods are based on the random vibration approach and thus, provide a statistical measure of the response, not controlled by a particular set of selected ground motions. In contrast with the random vibration approach that remains rather uncommon in engineering practice, response spectrum methods are particularly appealing from a design standpoint. The Multiple Support Response Spectrum (MSRS) method (Der Kiureghian and Neuenhofer, 1992), obtains the mean of the peak structural response in terms of the response spectra and peak ground displacements at the support points of the structure and the coherency function characterizing the ground motion spatial variability. This method properly accounts for cross-correlations that occur between the support motions and between modal responses. However, as a method employing modal superposition, the MSRS method is necessarily limited to linear analysis. One way to account for non-linear behavior is to conduct Response History Analysis (RHA) for specified time histories of ground motions at the support points of the structure. Since recordings of closely spaced earthquake motions are rare, non-linear RHA of multiply supported structures must rely on synthetic ground motions. For both approaches described above, analysis tools are developed and comprehensive parametric studies enhance the understanding of bridge response to spatially varying excitations. More details are given in the following.
Main developments and findings
Response spectrum method
Generalization and extension of the MSRS method: The original MSRS formulation only considered response quantities that could be expressed as linear combinations of the displacements at the unconstrained degrees of freedom (DOF) of the structure. The generalized formulation also allows consideration of response quantities that involve one or more support DOFs, e.g., drifts of bridge pier (Konakli and Der Kiureghian, 2011). The extended version of the MSRS rule accounts for the quasi-static contribution of truncated high-frequency modes and achieves improved approximations with small additional computational effort (Konakli and Der Kiureghian, 2011).
Computer implementation of the MSRS method: A computer algorithm for the implementation of the generalized and extended MSRS method is developed (Konakli and Der Kiureghian, 2011). In this algorithm, the evaluation of the cross-modal, cross-support correlation coefficients is performed with a numerical integration method that accounts for the behavior of the integrand for specific modal quantities. The computational efficiency achieved with this integration method is particularly important due to the large number of correlation coefficients involved in the MSRS analysis of typical bridge models. Another advantage of the implemented algorithm is the ability to evaluate the required response coefficients with a method that can be applied with any "black-box" structural analysis software with restricted access to the source code.
Parametric studies: Comparative response analyses of four real bridges designed by the California Department of Transportation (CalTrans) allow insightful observations on the influence of differential support motions on the structural response. Total, pseudo-static and dynamic responses are investigated for three cases of excitation: uniform support motions (case 1), varying support motions due only to wave passage and incoherence (case 2), and varying support motions due to wave passage, incoherence and differential site effect (case 3). The effect of spatial variability is found to be more significant on element forces along the deck and relatively mild on pier drifts. Among the bridges examined, the effect of spatial variability is more pronounced for the stiffer ones. For those bridges, additional variation of support soil properties causes large amplifications of many pseudo-static responses and, consequently, of the respective total responses (Konakli and Der Kiureghian, 2011).

Figure 3: Auburn Ravine Bridge: Mean peak element forces along the deck
Figure 4: Auburn Ravine Bridge: Relative pseudo-static contributions to mean peak element forces along the deck
RHA method
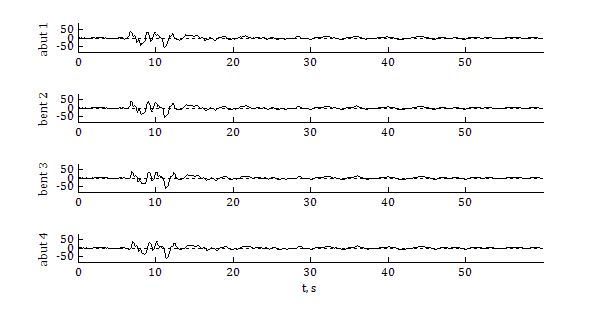
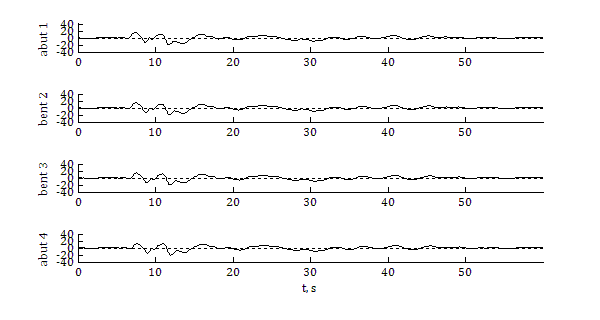
Synthetic motions for RHA: Methods for simulation of non-stationary spatially varying ground motion arrays incorporating the effects of incoherence, wave passage and differential site response are developed (Konakli and Der Kiureghian, 2011). The simulated motions inherit statistical characteristics of a specified acceleration record at a reference site. The conditional simulation approach preserves time-history characteristics of the specified record; however, the array of motions exhibits increasing variability with distance from the reference site. The unconditional simulation method generates an array of motions that preserve the overall temporal and spectral characteristics of the specified record and exhibit uniform variability at all locations. The simulated motions are validated by examining their physical compliance and by comparing their response spectra, coherency characteristics and power spectral densities with corresponding target models. By incorporating the differential site-response effect, the present work provides an important enhancement of the previously developed methods of conditional simulation (Vanmarcke and Fenton, 1991; Kameda and Morikawa, 1992; Liao and Zerva, 2006).
Acceleration time-histories (g)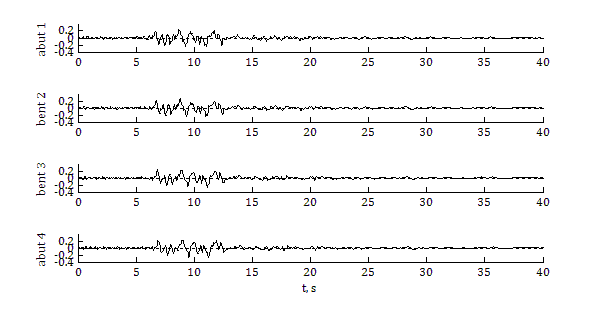
Velocity time-histories (cm/sec)
|
Displacement time-histories (cm)
|
Investigation of “equal displacement rule”: Time-history analyses of the same four bridge models used in the MSRS analysis are performed using as input ensembles of support motion arrays simulated with the unconditional approach. The effect of spatial variability on the “equal displacement” rule (Veletsos and Newmark, 1960) is investigated by examining the means and standard deviations of the ratios of peak non-linear over peak linear drifts (Konakli and Der Kiureghian, 2011).
case 1: uniform excitations
|

case 2: spatially varying excitations with incoherence and wave passage
|
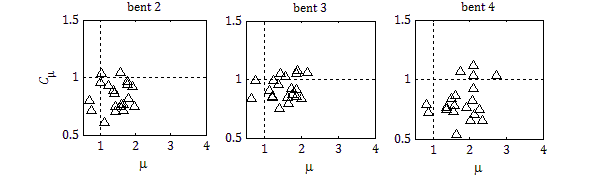
Figure 7: Ratio of non-linear to linear pier drifts, Cµ, versus ductility for the Penstock Bridge.
|
It is shown that under uniform soil conditions, spatial variability tends to decrease these ratios. The effect is more pronounced for stiffer bridges and smaller ductility ratios. When softer soils are considered, these ratios are increased. Similar to the case of uniform support excitations, the “equivalent displacement” rule gives fairly good approximations of the non-linear pier drifts for cases when the fundamental period of the bridge was larger than the predominant period of the site.
Accuracy of the MSRS method
As with other response spectrum methods, the MSRS rule involves fundamental assumptions and approximations rooted in the theory of random vibrations. These include the assumptions that the ground motion is a broadband process with a strong motion duration several times longer than the fundamental period of the structure and that the spatial variability of the ground motion random field is described by a smooth coherency function. The error encountered by use of MSRS is unknown and can be on either conservative or unconservative side. Comparisons between mean peak responses obtained from linear RHA and MSRS analyses provide information on the range of errors induced by the approximations involved in the latter method (Konakli and Der Kiureghian, 2011).
Coherency analysis
In all analysis approaches discussed above, a coherency function is typically employed to describe the spatial variability of support motions. Because of the random nature of the incoherence component of this function, it is not possible to develop a theoretical model of this phenomenon solely based on physics. A detailed coherency analysis of the near-fault recordings of the UPSAR array from the 2004 Parkfield (California) earthquake assesses the ability of commonly used empirical models (e.g. Abrahamson et al., 1991) and semi-empirical models (e.g. Luco and Wong, 1986 ) to describe the incoherence component of this array (Konakli and Der Kiureghian, 2011).
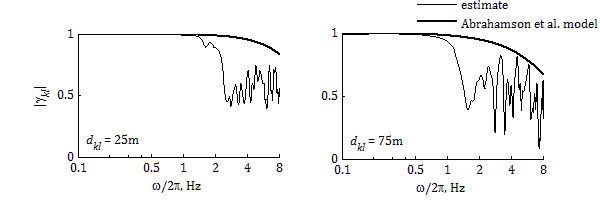
Figure 8: Comparison of horizontal coherency estimates from the UPSAR array with the Abrahamson et al. model
References
Abrahamson NA, Schneider JF, Stepp JC. Empirical spatial coherency functions for application to soil-structure interaction analyses. Earthquake Spectra 1991; 7:1-28.
Der Kiureghian A. A coherency model for spatially varying ground motions. Earthquake Engineering and Structural Dynamics 1996; 25:99-111.
Der Kiureghian A, Neuenhofer A. Response spectrum method for multiple-support seismic excitation. Earthquake Engineering and Structural Dynamics 1992; 21:713-740.
Konakli K. and Der Kiureghian A. Extended MSRS rule for seismic analysis of bridges subjected to differential support motions. Earthquake Engineering and Structural Dynamics 2011; 40:315-335.
Konakli K. and Der Kiureghian A. Simulation of spatially varying ground motions including incoherence, wave-passage and differential site-response effects. Earthquake Engineering and Structural Dynamics 2011 (published online)
Konakli K. and Der Kiureghian A. Stochastic dynamic analysis of bridges subjected to spatially varying ground motions. Report No. UCB/EERC-2011/105, Earthquake Engineering Research Center, University of California, Berkeley, 2011.
Liao S, Zerva A. Physically compliant, conditionally simulated spatially variable seismic ground motions for performance-based design. Earthquake Engineering and Structural Dynamics 2006; 35:891-919.
Luco JE, Wong HL. Response of a rigid foundation to a spatially random ground motion. Earthquake Engineering and Structural Dynamics 1986; 14:891-908.
Kameda H, Morikawa H. An interpolating stochastic process for simulation of conditional random fields. Probabilistic Engineering Mechanics 1992; 7:243-254.
Vanmarcke EH, Fenton GA. Conditioned simulation of local fields of earthquake ground motion. Structural Safety 1991; 10:247-264.
Veletsos AS, Newmark NM. Effect of inelastic behavior on the response of simple systems to earthquake motions. Proceedings of the 2nd World Conference on Earthquake Engineering, Japan, 2:895-912, 1960.